Archive for the ‘mathematics’ category: Page 151
Jul 11, 2016
Stanford University Researchers Help DARPA Create Mathematical Framework for Network Pattern Discovery
Posted by Karen Hurst in categories: engineering, information science, mathematics
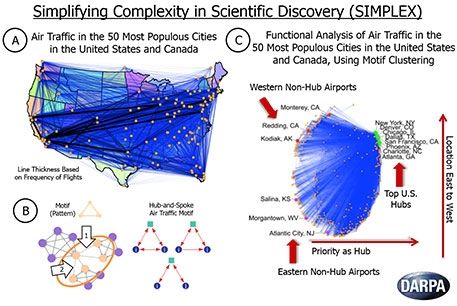
The Defense Advanced Research Projects Agency has demonstrated a new mathematical framework that works to help researchers discover patterns in complex scientific and engineering systems. DARPA said Thursday researchers at Stanford University created algorithms designed to explore patterns in data in order to gain insights into network structure and function under the Simplifying Complexity in Scientific Discovery [ ].
Jul 9, 2016
Fighting ISIS With an Algorithm, Physicists Try to Predict Attacks
Posted by Aleksandar Vukovic in categories: information science, mathematics, physics
A new mathematical model aims to track the activity of Islamic State sympathizers online and determine when groups will turn from talk to action.
Jul 9, 2016
Mathematical framework that prioritizes key patterns in networks aims to accelerate scientific discovery
Posted by Karen Hurst in categories: biological, finance, information science, mathematics, military
Nice.
Networks are mathematical representations to explore and understand diverse, complex systems—everything from military logistics and global finance to air traffic, social media, and the biological processes within our bodies. In each of those systems, a hierarchy of recurring, meaningful internal patterns—such as molecules and proteins interacting inside cells, and capacitors and resistors operating within integrated circuits—determines the functions or behaviors of those systems. The larger and more intricate a system is, however, the harder it is for current network modeling techniques to uncover these patterns and represent them in organized, easy-to-understand ways.
Researchers at Stanford University, funded by DARPA’s Simplifying Complexity in Scientific Discovery (SIMPLEX) program, have made progress in overcoming these challenges through a framework they have developed for identifying and clustering what mathematicians call “motifs”: essential but often obscure patterns within systems that are the building blocks of mathematical modeling and that facilitate the computational representation of complex systems.
Jul 2, 2016
MND: Your Daily Dose of Counter-Theory
Posted by Karen Hurst in categories: mathematics, neuroscience
Thomas Aquinas and other ludicrous pseudo-philosophers (in contradistinction with real philosophers such as Abelard) used to ponder questions about angels, such as whether they can interpenetrate (as bosons do).
Are today’s mathematicians just as ridiculous? The assumption of infinity has been “proven” by the simplest reasoning ever: if n is the largest number, clearly, (n+1) is larger. I have long disagreed with that hare-brained sort of certainty, and it’s not a matter of shooting the breeze. (My point of view has been spreading in recent years!) Just saying something exists, does not make it so (or then one would believe Hitler and Brexiters). If I say:” I am emperor of the galaxy known as the Milky Way!” that has a nice ring to it, but it does not make it so (too bad, that would be fun).
Given n symbols, each labelled by something, can one always find a new something to label (n+1) with? I say: no. Why? Because reality prevents it. Somebody (see below) objected that I confused “map” and “territory”. But I am a differential geometer, and the essential idea there, from the genius B. Riemann, is that maps allow to define “territory”:
Jun 25, 2016
New analog compiler could help enable simulation of whole organs and even organisms
Posted by Shailesh Prasad in categories: biotech/medical, computing, mathematics
A transistor, conceived of in digital terms, has two states: on and off, which can represent the 1s and 0s of binary arithmetic.
But in analog terms, the transistor has an infinite number of states, which could, in principle, represent an infinite range of mathematical values. Digital computing, for all its advantages, leaves most of transistors’ informational capacity on the table.
In recent years, analog computers have proven to be much more efficient at simulating biological systems than digital computers. But existing analog computers have to be programmed by hand, a complex process that would be prohibitively time consuming for large-scale simulations.
Jun 22, 2016
White House issues report on President Obama’s impact on science and tech
Posted by Karen Hurst in categories: biotech/medical, education, mathematics, policy, science
In 2009, President Obama pledged to “restore science to its rightful place.” He said, “We will not just meet, but we will exceed the level achieved at the height of the space race, through policies that invest in basic and applied research, create new incentives for private innovation, promote breakthroughs in energy and medicine, and improve education in math and science.”
Today, the White House released an Impact Report listing 100 things that Obama has made happen with the support of many people across research, policy, education, and, yes, maker culture. Here’s the full Impact Report. A few examples from the list:
Jun 15, 2016
Did gravitational wave detector find dark matter?
Posted by Andreas Matt in categories: cosmology, information science, mathematics, physics
When an astronomical observatory detected two black holes colliding in deep space, scientists celebrated confirmation of Einstein’s prediction of gravitational waves. A team of astrophysicists wondered something else: Had the experiment found the “dark matter” that makes up most of the mass of the universe?
The eight scientists from the Johns Hopkins Henry A. Rowland Department of Physics and Astronomy had already started making calculations when the discovery by the Laser Interferometer Gravitational-Wave Observatory (LIGO) was announced in February. Their results, published recently in Physical Review Letters, unfold as a hypothesis suggesting a solution for an abiding mystery in astrophysics.
“We consider the possibility that the black hole binary detected by LIGO may be a signature of dark matter,” wrote the scientists in their summary, referring to the black hole pair as a “binary.” What follows are five pages of annotated mathematical equations showing how the researchers considered the mass of the two objects LIGO detected as a point of departure, suggesting that these objects could be part of the mysterious substance known to make up about 85 percent of the mass of the universe.
Jun 13, 2016
A classic formula for pi has been discovered hidden in hydrogen atoms
Posted by Andreas Matt in categories: mathematics, particle physics, quantum physics
For the first time, scientists have discovered a classic formula for pi in the world of quantum physics. Pi is the ratio between a circle’s circumference and its diameter, and is incredibly important in pure mathematics, but now scientists have also found it “lurking” in the world of physics, when using quantum mechanics to compare the energy levels of a hydrogen atom.
Why is that exciting? Well, it reveals an incredibly special and previously unknown connection between quantum physics and maths.
“I find it fascinating that a purely mathematical formula from the 17th century characterises a physical system that was discovered 300 years later,” said one of the lead researchers, Tamar Friedmann, a mathematician at the University of Rochester in the US. Seriously, wow.
Continue reading “A classic formula for pi has been discovered hidden in hydrogen atoms” »
Jun 8, 2016
Worldwide quantum web may be possible with help from graphs
Posted by Karen Hurst in categories: internet, mathematics, particle physics, quantum physics, security
(Phys.org)—One of the most ambitious endeavors in quantum physics right now is to build a large-scale quantum network that could one day span the entire globe. In a new study, physicists have shown that describing quantum networks in a new way—as mathematical graphs—can help increase the distance that quantum information can be transmitted. Compared to classical networks, quantum networks have potential advantages such as better security and being faster under certain circumstances.
“A worldwide quantum network may appear quite similar to the internet—a huge number of devices connected in a way that allows the exchange of information between any of them,” coauthor Michael Epping, a physicist at the University of Waterloo in Canada, told Phys.org. “But the crucial difference is that the laws of quantum theory will be dominant for the description of that information. For example, the state of the fundamental information carrier can be a superposition of the basis states 0 and 1. By now, several advantages in comparison to classical information are known, such as prime number factorization and secret communication. However, the biggest benefit of quantum networks might well be discovered by future research in the rapidly developing field of quantum information theory.”
Quantum networks involve sending entangled particles across long distances, which is challenging because particle loss and decoherence tend to scale exponentially with the distance.